

About Me
I am a Stevenson Professor and Department Chair in the Department of Mathematics at Vanderbilt University. A long time ago I attended Mount Olive High School in Flanders, New Jersey. I was an undergraduate at Brown University, where I worked with Thomas Banchoff. I graduated with a Ph.D. from the University of Chicago in 2003. My thesis advisor was Benson Farb. I did an NSF postdoc at the University of Utah with Mladen Bestvina from 2003 to 2008. I then spent two years as an Assistant Professor at Tufts University and 13 years at Georgia Institute of Technology before taking my current position. I received an NSF Career Award and a Sloan Fellowship, and was the inaugural recipient of the Leddy Family Faculty Fellowship at Georgia Tech. I am a Fellow of the American Mathematical Society, inducted in 2019 "for contributions to low-dimensional topology and geometric group theory, exposition, and mentoring." I received the 2021 Levi Conant Prize from the AMS for my article about the mathematics of Joan Birman, and gave the 2022 Levi L. Conant Lecture. I was the Maryam Mirzakhani Lecturer at the 2022 Joint Mathematics Meetings (video). In 2024 Benson Farb and I were the co-recipients of the Leroy P. Steele Prize for Mathematical Exposition from the American Mathematical Society, in recognition of our book, A Primer on Mapping Class Groups. For more on my career, see my Curriculum Vita or this interview.My research is in the intersection of low-dimensional topology and geometric group theory. I am mainly interested in mapping class groups of surfaces, or, the study of surfaces and their symmetries. This subject is exciting because it connects to - and uses ideas from - many areas of mathematics, including surface bundles, dynamics, representation theory, number theory, hyperbolic geometry, Teichmuller theory, and algebraic geometry.
Surfaces and their symmetries
A surface is a two-dimensional shape. Here are some:

|

|

|

|
There are lots of symmetries that are easy to find: the sphere and the torus have infinitely many rotational symmetries. The double torus and the triple torus also admit rotational symmetries, but only finitely many. For instance the double torus admits a rotation by π and the triple torus admits a rotation by 2π/3.
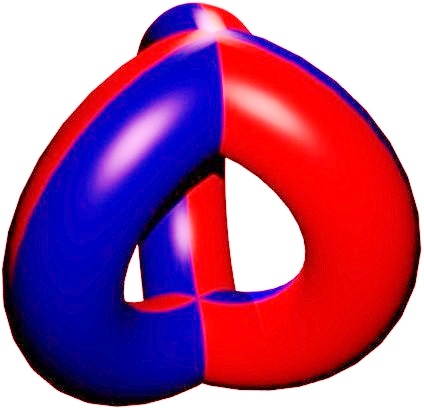 There are other symmetries that are more subtle. For instance, the double torus has a symmetry that is a 2π/3 rotation! Here is the trick: in topology we say two objects are the same if we can deform one into the other without cutting or gluing). The surface shown on the right (by Matthias Goerner) is the same as the double torus (can you see how to deform one into the other?). Since the surface on the right has a rotational symmetry with angle 2π/3, the original double torus does as well. Here is a cute video playing off of the same idea.
There are other symmetries that are more subtle. For instance, the double torus has a symmetry that is a 2π/3 rotation! Here is the trick: in topology we say two objects are the same if we can deform one into the other without cutting or gluing). The surface shown on the right (by Matthias Goerner) is the same as the double torus (can you see how to deform one into the other?). Since the surface on the right has a rotational symmetry with angle 2π/3, the original double torus does as well. Here is a cute video playing off of the same idea.
The set of all symmetries of a surface (modulo homotopy, or, deformation) is known as the mapping class group of the surface. This is a group because we can multiply two elements by composing them. My research centers on the mapping class group and its variations, such as braid groups and automorphism groups of free groups. My work generally falls into two categories:
- What can we say about the algebraic structure of the mapping class group as a whole?
- What can we say about the dynamics of individual elements of the mapping class group?
Algebraic structure of the mapping class group
 An example of an answer to the first question is the theorem of Dehn from the 1920s that the mapping class group is generated by Dehn twists. A Dehn twist is a symmetry of a surface obtained by cutting the surface along a simple closed curve (a circle in the surface), twisting one side by 2π and then regluing. You might protest at this point because I said before that cutting and gluing are not allowed. But since we twisted by exactly 2π, points that were close before the operation are still close afterwards (in other words we have defined a continuous function). The sequence of pictures on the right demonstrates the effect on the curve γ of the Dehn twist about the curve α.
An example of an answer to the first question is the theorem of Dehn from the 1920s that the mapping class group is generated by Dehn twists. A Dehn twist is a symmetry of a surface obtained by cutting the surface along a simple closed curve (a circle in the surface), twisting one side by 2π and then regluing. You might protest at this point because I said before that cutting and gluing are not allowed. But since we twisted by exactly 2π, points that were close before the operation are still close afterwards (in other words we have defined a continuous function). The sequence of pictures on the right demonstrates the effect on the curve γ of the Dehn twist about the curve α.
Unlike our previous example of symmetries, a Dehn twist has infinite order: if you keep doing it, the surface gets more and more twisted and never returns to its starting state. We can think of a Dehn twist as a Rubik's cube move for topologists. It is remarkable that every symmetry of a surface can be obtained from a sequence of these moves. Even the rotational symmetries we saw before are products of Dehn twists - that is pretty hard to imagine! And, as we will see below, there are very complicated symmetries to consider.
We can think of a Dehn twist as a Rubik's Cube-type move on the surface. If you like that idea, try out the game Teruaki, written by Kazushi Ahara, by clicking here.
In my research with Brendle and Putman, we found a generating set for a certain subgroup of the mapping class group called the hyperelliptic Torelli group. Again the generating set consists of Dehn twists, but only certain kinds (separating curves that are preserved by the hyperelliptic involution). This theorem settled a algebro-geometric conjecture of Dick Hain about a certain moduli space of curves.
My work with Bestvina and Bux gives the cohomological dimension of the Torelli subgroup of the mapping class group. For one thing, this theorem gives a way of seeing that certain abstract groups cannot be realized as subgroups of the Torelli group: if a group has larger dimension than the Torelli group - like a free abelian group of very large rank - then it cannot be isomorphic to a subgroup of the Torelli group.
Questions about the algebraic structure of the mapping class group very often reduce to questions about the combinatorics of simple closed curves in the surface. This is analogous to how we can understand a linear transformation through its action on vectors. Here is a complicated pair of curves that comes up in my work with Birman and Menasco:

My first paper with Brendle shows that the automorphism group of the Johnson kernel (an infinite index subgroup of the mapping class group) is isomorphic to the mapping class group. We can think of this result as saying that this infinite index subgroup remembers the algebraic structure of the full group. To prove this theorem, we reduce the problem to a problem about (separating) simple closed curves in the surface. Our current work amounts to a vast generalization of our first result.
Dynamics of individual elements of the mapping class group
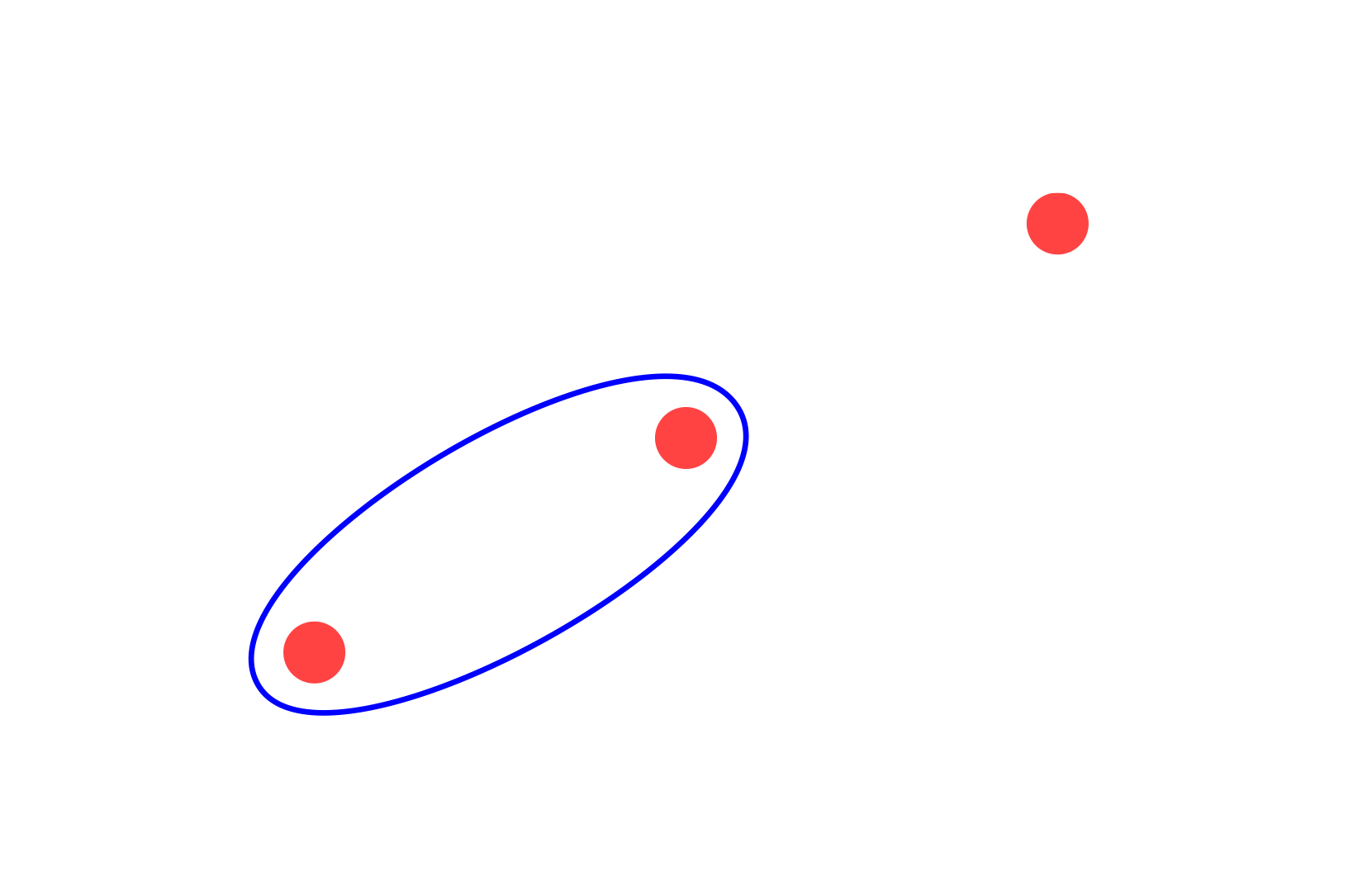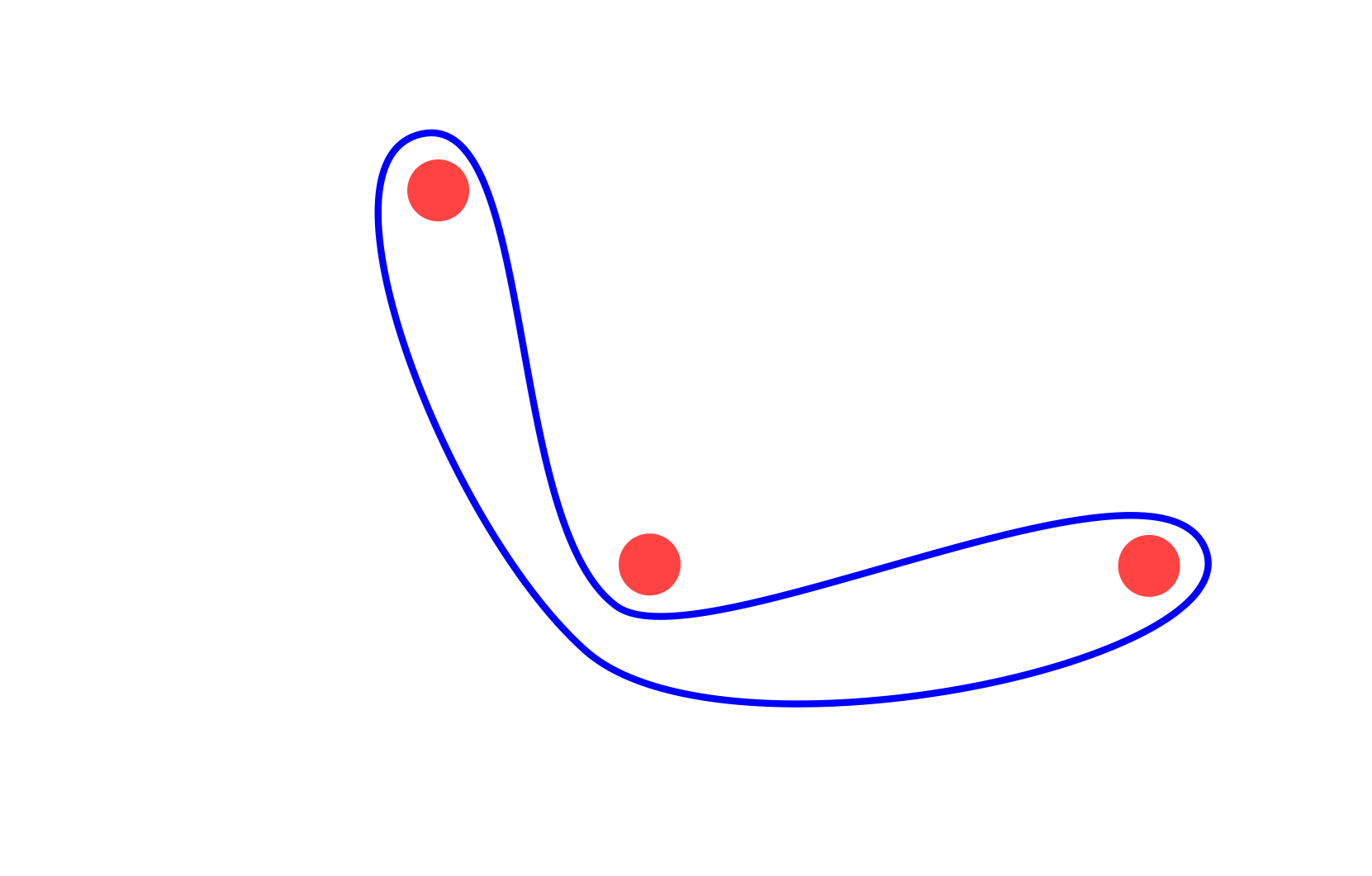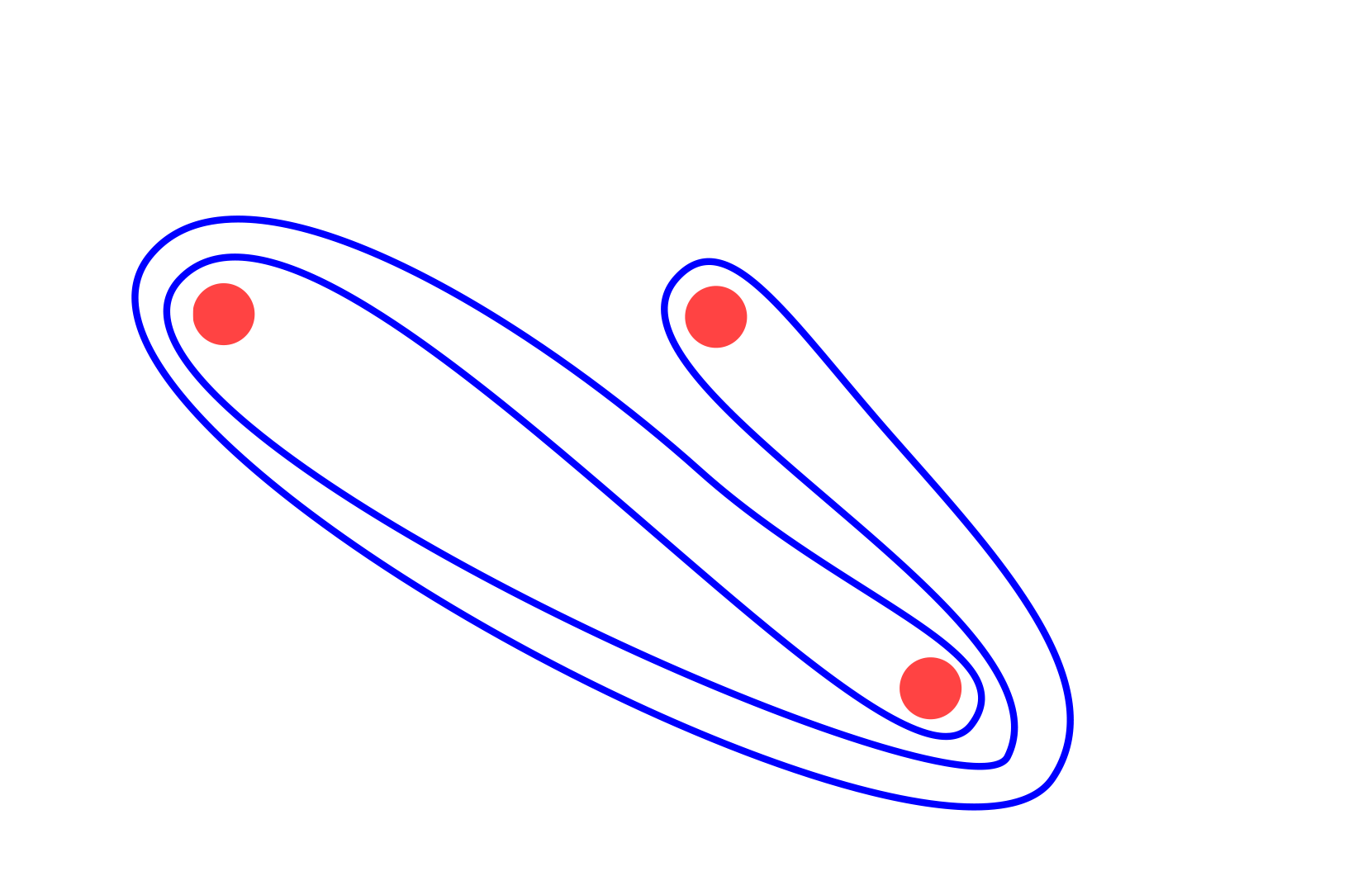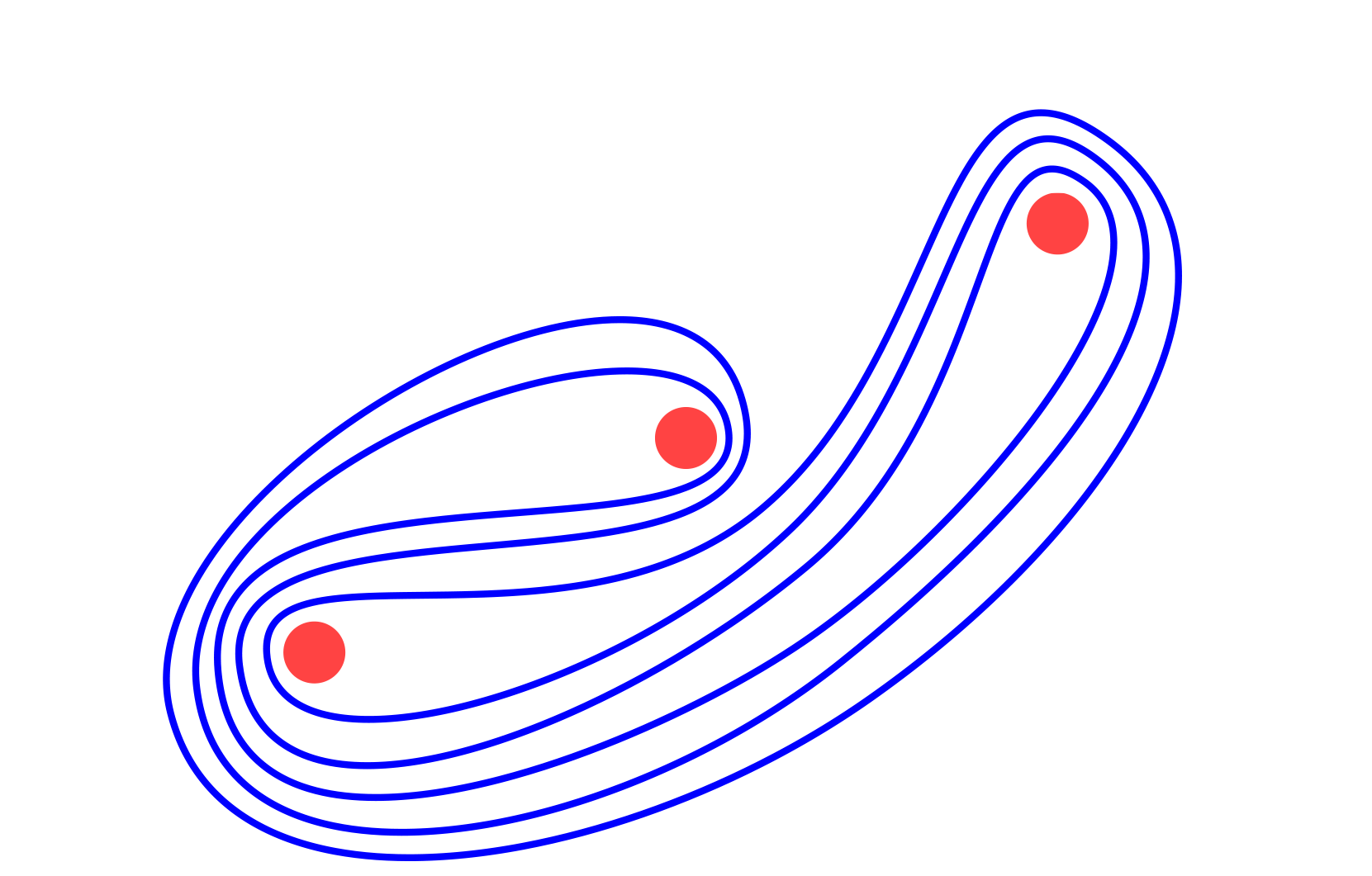
 Now let's talk about the second question. The idea here is to think of a symmetry of a surface as mixing the surface, and we want to understand this mixing. Consider for instance the three points moving around on the right. We can think of this motion as giving a symmetry of the plane with 3 marked points (the three red points). But how much mixing is being effected? Following ideas of the Fields medalist William Thurston, we can understand the mixing by looking at what it does to a curve in the plane. This is depicted in the animation below. You should think of this animation as showing a cross-section of a taffy puller.
Now let's talk about the second question. The idea here is to think of a symmetry of a surface as mixing the surface, and we want to understand this mixing. Consider for instance the three points moving around on the right. We can think of this motion as giving a symmetry of the plane with 3 marked points (the three red points). But how much mixing is being effected? Following ideas of the Fields medalist William Thurston, we can understand the mixing by looking at what it does to a curve in the plane. This is depicted in the animation below. You should think of this animation as showing a cross-section of a taffy puller.
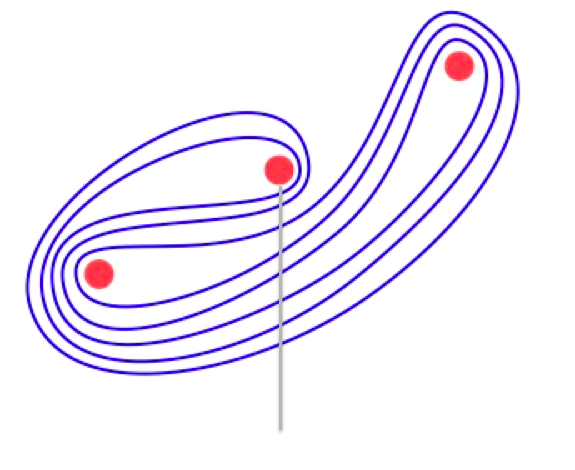
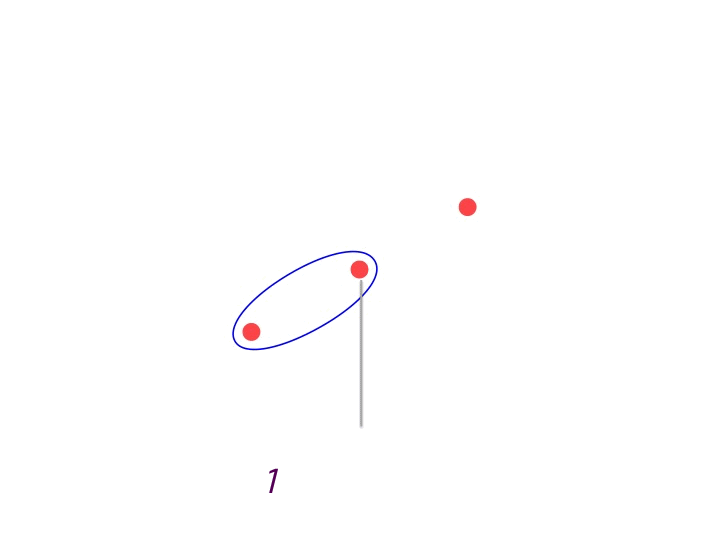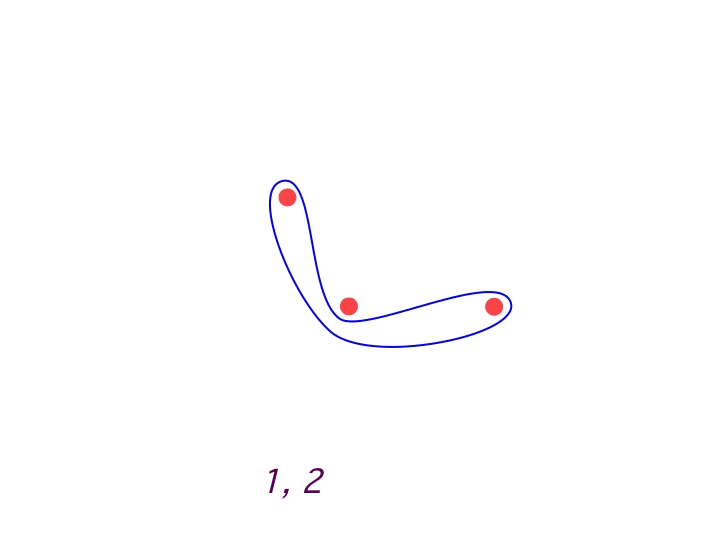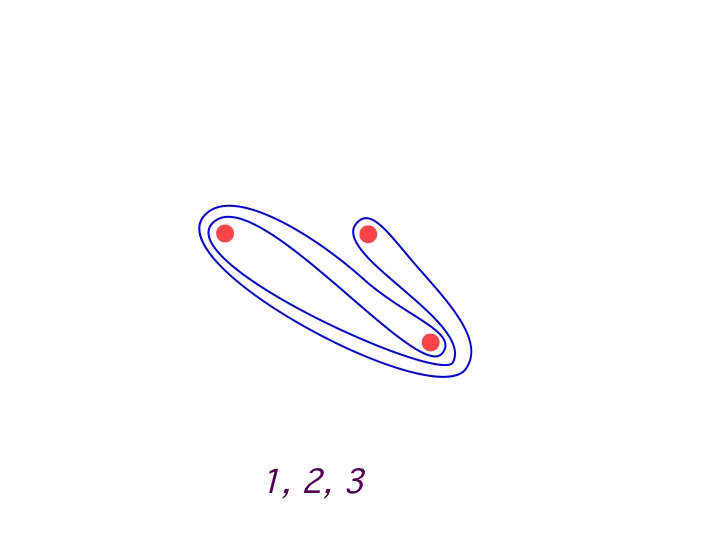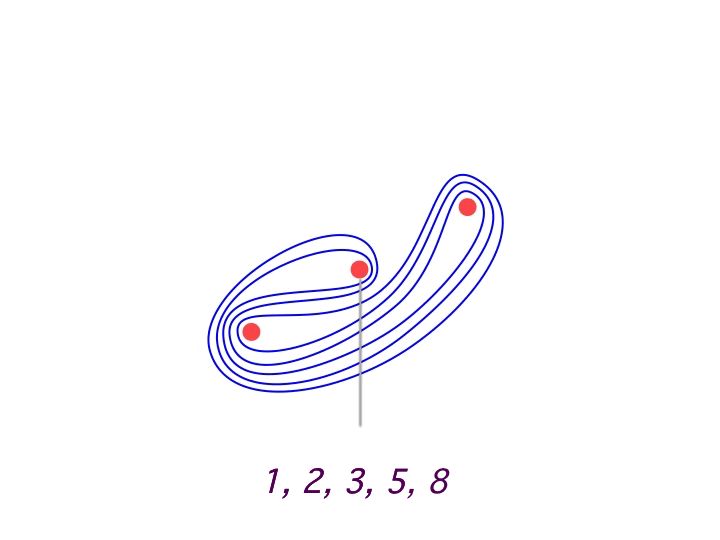
In the 1970s Thurston developed a general theory for understanding these taffy puller-type symmetries - which he called pseudo-Anosov homeomorphisms - and for computing their stretch factors. My paper with Farb and Leininger gives a structure to the set of pseudo-Anosov homeomorphisms with small stretch factor. There are infinitely many such pseudo-Anosov homeomorphisms (if we let the surface vary). We show that, in a certain precise sense, that all of these are born from a finite list of 3-manifolds. My current work with Strenner and Yurttas gives a very fast (quadratic time) algorithm for computing stretch factors of pseudo-Anosov homeomorphisms.
Epilogue
Dehn started work on the mapping class group in the 1920s - almost a century ago. Still, there are basic questions that remain unanswered: Does every mapping class group admit a faithful representation to some general linear group over the complex numbers? Is there a finite index subgroup of the mapping class group with infinite abelianization? What is the structure of the cohomology of the mapping class group? Is the Torelli group finitely presented? What is the smallest stretch factor on a triple torus? Which real numbers are stretch factors?My research is devoted to understanding these and related questions. If you'd like to learn more about mapping class groups, you can read the book I wrote with Farb, A Primer on Mapping Class Groups or the chapter I wrote with Brendle and Childers in Office Hours with a Geometric Group Theorist. Or contact me directly. I hope you are inspired to look more into these topics and that you enjoy them as much as I do!